Let us draw the figure as below –

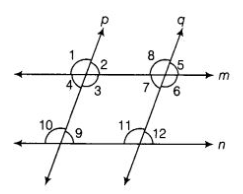
It is given to us that there are two parallel lines. Let us assume l and m are two parallel lines, i.e., l || m.

Also, it is given that two lines are perpendicular to the parallel lines. Let us assume n and p are the two perpendicular lines.

⇒ n ⊥ l, n ⊥ m, p ⊥ l, and p ⊥ m

Let us assume the angles as ∠1, ∠2, …, ∠16 as shown in the figure.

To show that n and p are parallel to each other, i.e., to prove n || p.

We know that l || m, n ⊥ l, and n ⊥ m.

⇒ ∠1 = ∠2 = ∠3 = ∠4 = 90°, and ∠9 = ∠10 = ∠11 = ∠12 = 90°

⇒ ∠1 = ∠9 = 90°, ∠2 = ∠10 = 90° (Corresponding angles)

Similarly, ∠4 = ∠10 = 90°, ∠3 = ∠9 = 90° (Alternate interior angles)

Again, l || m, p ⊥ l, and p ⊥ m.

⇒ ∠5 = ∠6 = ∠7 = ∠8 = 90°, and ∠13 = ∠14 = ∠15 = ∠16 = 90°

We have ∠3 = 90° and ∠8 = 90°

⇒ ∠3 + ∠8 = 90° + 90° = 180°

Thus, we can say that the sum of the two interior angles is supplementary.

We know, if a transversal intersects two lines, such that each pair of interior angles on the same side of the transversal is supplementary, then the two lines are parallel to each other.

Thus, n || p.