In Fig. 6.39, sides QP and RQ of Δ PQR are produced to points S and T respectively. If ∠ SPR = 135° and ∠ PQT = 110°, find ∠ PRQ.
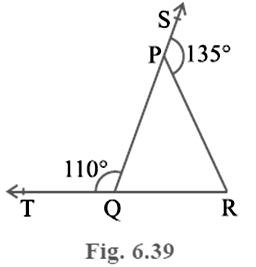
It is given in the question that:
∠SPR = 135O
And,
∠PQT = 110o
Now, according to the question,
∠SPR + ∠QPR = 180O (SQ is a straight line)
135o + ∠QPR = 180O
∠QPR = 45O
And,
∠PQT + ∠PQR = 180O (TR is a straight line)
110o + ∠PQR = 180O
∠PQR = 70O
Now,
∠PQR + ∠QPR + ∠PRQ = 180O (Sum of the interior angles of the triangle)
70o + 45o + ∠PRQ = 180O
115O + ∠PRQ = 180O
∠PRQ = 65O
23