In Fig. 6.40, ∠ X = 62°, ∠ XYZ = 54°. If YO and ZO are the bisectors of ∠ XYZ and∠ XZY respectively of Δ XYZ, find ∠ OZY and ∠ YOZ.

Question

In Fig. 6.40, &#x2220; X = 62&#xB0;, &#x2220; XYZ = 54&#xB0;. If YO and ZO are the bisectors of &#x2220; XYZ and &#x2220; XZY respectively of &#x394; XYZ, find &#x2220; OZY and &#x2220; YOZ.

Philoid · Accepted Answer

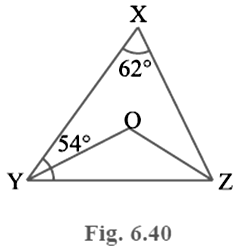
It is given in the question that:

∠X = 62^o, ∠XYZ = 54^o

YO and ZO are the bisectors of ∠XYZ and ∠XZY respectively.

Now, according to the question,

∠X + ∠XYZ + ∠XZY = 180^o (Sum of the interior angles of triangle)

62^o + 54^o + ∠XZY = 180^o

116^o + ∠XZY = 180^o

∠XZY = 64^o

Now,

∠OZY =

∠OZY = 32^o

And,

∠OYZ = ∠XYZ (YO is the bisector)

∠OYZ = 27^o

Now,

∠OZY + ∠OYZ + ∠O = 180^o (Sum of the interior angles of the triangle)

32^o + 27^o + ∠O = 180^o

59^o + ∠O = 180^o

∠O = 121^o