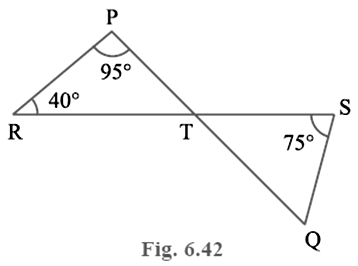
In Fig. 6.42, if lines PQ and RS intersect at point T, such that ∠ PRT = 40°, ∠ RPT = 95°and ∠ TSQ = 75°, find ∠ SQT.

Question

In Fig. 6.42, if lines PQ and RS intersect at point T, such that &#x2220; PRT = 40&#xB0;, &#x2220; RPT = 95&#xB0;and &#x2220; TSQ = 75&#xB0;, find &#x2220; SQT.

Philoid · Accepted Answer

It is given in the question that:

∠PRT = 40^o

∠RPT = 95^o and,

∠TSQ = 75^o

Now according to the question,

∠PRT + ∠RPT + ∠PTR = 180^o (Sum of interior angles of the triangle)

40^o + 95^o + ∠PTR = 180^o

135^o + ∠PTR = 180^o

∠PTR = 45^o

∠PTR = ∠STQ = 45^o (Vertically opposite angles)

Now,

∠TSQ + ∠PTR + ∠SQT = 180^o (Sum of the interior angles of the triangle)

75^o + 45^o + ∠SQT = 180^o

120^o + ∠SQT = 180^o

∠SQT = 60^o