Line l is the bisector of an angle ∠ A and B is any point on l. BP and BQ are perpendiculars from B to the arms of ∠ A (see Fig. 7.20). Show that: (i) Δ APB ≅ Δ AQB (ii) BP = BQ or B is equidistant from the arms of ∠ A.

Question

Line l is the bisector of an angle &#x2220; A and B is any point on l. BP and BQ are perpendiculars from B to the arms of &#x2220; A (see Fig. 7.20). Show that: (i) &#x394; APB &#x2245; &#x394; AQB (ii) BP = BQ or B is equidistant from the arms of &#x2220; A.

Philoid · Accepted Answer

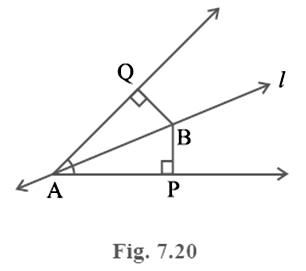
It is given in the question that:

l is the bisector of an ∠A

BP and BQ are perpendiculars

(i) In

∠P = ∠Q (Right angles)

∠BAP = ∠BAQ (l is the bisector)

AB = AB (Common)

Therefore,

By AAS congruence,

(ii) BP = BQ (By c.p.c.t)

Therefore,

B is equidistant from the arms of ∠A

Line l is the bisector of an angle ∠ A and B is any point on l. BP and BQ are perpendiculars from B to the arms of ∠ A (see Fig. 7.20). Show that:(i) Δ APB ≅Δ AQB(ii) BP = BQ or B is equidistant from the arms of ∠ A.

Line l is the bisector of an angle ∠ A and B is any point on l. BP and BQ are perpendiculars from B to the arms of ∠ A (see Fig. 7.20). Show that:
(i) Δ APB ≅Δ AQB

(ii) BP = BQ or B is equidistant from the arms of ∠ A.