In an isosceles triangle ABC, with AB = AC, the bisectors of ∠ B and ∠ C intersect each other at O. Join A to O. Show that:

Question

In an isosceles triangle ABC, with AB = AC, the bisectors of &#x2220; B and &#x2220; C intersect each other at O. Join A to O. Show that: (i) OB = OC (ii) AO bisects &#x2220; A

Philoid · Accepted Answer

It is given in the question that:

AB = AC

The bisectors of ∠B and ∠C intersect each other at O

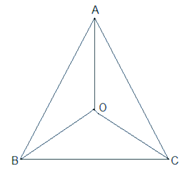
(i) ABC is an isosceles with AB = AC

Therefore,

∠B = ∠C

∠OBC = ∠OCB (Angles bisectors)

OB = OC (Side opposite to the equal angles are equal)

(i) In

AB = AC (Given)

AO = AO (Common)

OB = OC (Proved above)

Therefore,

By SSS congruence rule

∠BAO = ∠CAO (By c.p.c.t)

Thus,

AO bisects ∠A

In an isosceles triangle ABC, with AB = AC, the bisectors of ∠ B and ∠ C intersect each other at O. Join A to O. Show that:(i) OB = OC(ii) AO bisects ∠ A

In an isosceles triangle ABC, with AB = AC, the bisectors of ∠ B and ∠ C intersect each other at O. Join A to O. Show that:
(i) OB = OC

(ii) AO bisects ∠ A