ΔABC is an isosceles triangle in which AB = AC. Side BA is produced to D such that AD = AB (see Fig. 7.34). Show that ∠ BCD is a right angle.

Question

&#x394;ABC is an isosceles triangle in which AB = AC. Side BA is produced to D such that AD = AB (see Fig. 7.34). Show that &#x2220; BCD is a right angle.

Philoid · Accepted Answer

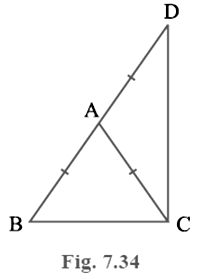
It is given in the question that:

AB = AC and

AD = AB

Proof: In

AB = AC (Given)

∠ACB = ∠ABC (Angles opposite to equal sides are equal)

In

AD = AB

∠ADC = ∠ACD (Angles opposite to equal sides are equal)

Now,

In

∠CAB + ∠ACB + ∠ABC = 180^o

∠CAB + 2∠ACB = 180^o

∠CAB = 180^o - 2∠ACB (i)

Similarly,

In

∠CAD = 180^o - 2∠ACD (ii)

Also,

∠CAB + ∠CAD = 180^o (BD is a straight line)

Adding (i) and (ii), we get

∠CAB + ∠CAD = 180^o - 2∠ACB + 180^o - 2∠ACD

180^o = 360^o - 2∠ACB - 2∠ACD

2 (∠ACB + ∠ACD) = 180^o

∠BCD = 90^o