AD is an altitude of an isosceles triangle ABC in which AB = AC. Show that (i) AD bisects BC (ii) AD bisects ∠ A

Question

AD is an altitude of an isosceles triangle ABC in which AB = AC. Show that (i) AD bisects BC (ii) AD bisects &#x2220; A

Philoid · Accepted Answer

It is given in the question that:

AD is an altitude and AB = AC

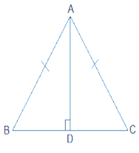
(i) In

∠ADB = ∠ADC = 90^o

AB = AC (Given)

AD = AD (Common)

Therefore,

By RHS axiom,

(By c.p.c.t)

(ii) ∠BAD = ∠CAD (By c.p.c.t)

Thus,

Ad bisects ∠A