BE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles.

Question

Philoid · Accepted Answer

It is given in the question that:

BE and CF are two equal altitudes

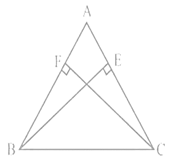
In

∠BEC = ∠CFB = 90^o (Altitudes)

BC = CB (Common)

BE = CF (Common)

Therefore,

By RHS axiom,

Now,

∠C = ∠B (By c.p.c.t)

Thus,

AB = AC as sides opposite to the equal angles are equal