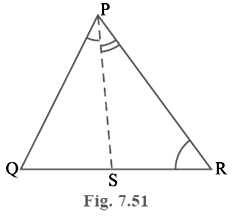
It is given in the question that:

PR > PQ and PS bisects ∠QPR

To prove: ∠PSR > PSQ

Proof: ∠PQR > ∠PRQ (i) (PR > PQ as angle opposite to larger side is larger)

∠QPS = ∠RPS (ii) (PS bisects ∠QPR)

∠PSR = ∠PQR + ∠QPS (iii) (Exterior angle of a triangle equals to the sum of opposite interior angles)

∠PSQ = ∠PRQ + ∠RPS (iv) (Exterior angle os a triangle equals to the sum of opposite interior angles)

Adding (i) and (ii), we get

∠PQR + ∠QPS > ∠PRQ + ∠RPS

∠PSR > ∠PSQ [From (i), (ii), (iii) and (iv)]