ABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD (see Fig. 8.21). Show that
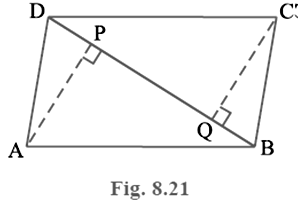
(i) Δ APB ≅Δ CQD
(ii) AP = CQ
(i) In ΔAPB and ΔCQD,
∠APB = ∠CQD (Each 90°)
AB = CD (Opposite sides of parallelogram ABCD)
∠ABP= ∠CDQ (Alternate interior angles for AB || CD)
ΔAPB ![]() ΔCQD (By AAS congruency)
ΔCQD (By AAS congruency)
(ii) By using the above result
ΔAPB ![]() ΔCQD, we obtain
ΔCQD, we obtain
AP = CQ (By CPCT)
42