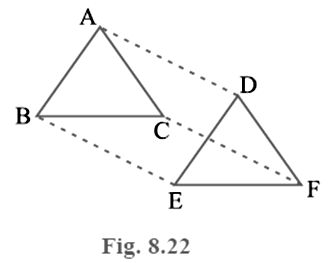
In Δ ABC and Δ DEF, AB = DE, AB || DE, BC = EF and BC || EF. Vertices A, B and C are joined to vertices D, E and F respectively (see Fig. 8.22). Show that (i) Quadrilateral ABED is a parallelogram (ii) Quadrilateral BEFC is a parallelogram (iii) AD || CF and AD = CF (iv) Quadrilateral ACFD is a parallelogram (v) AC = DF (vi) Δ ABC ≅ Δ DEF.

Question

In &#x394; ABC and &#x394; DEF, AB = DE, AB || DE, BC = EF and BC || EF. Vertices A, B and C are joined to vertices D, E and F respectively (see Fig. 8.22). Show that (i) Quadrilateral ABED is a parallelogram (ii) Quadrilateral BEFC is a parallelogram (iii) AD || CF and AD = CF (iv) Quadrilateral ACFD is a parallelogram (v) AC = DF (vi) &#x394; ABC &#x2245; &#x394; DEF.

Philoid · Accepted Answer

(i) Given that: AB = DE and

AB || DE

If two opposite sides of a quadrilateral are equal and parallel to each other, then it will be a parallelogram.

Therefore, quadrilateral ABED is a parallelogram

(ii) Again,

BC = EF and BC || EF

Therefore, quadrilateral BCEF is a parallelogram

(iii) As we had observed that ABED and BEFC are parallelograms

Therefore,

AD = BE and AD || BE

(Opposite sides of a parallelogram are equal and parallel)

And,

BE = CF and BE || CF

(Opposite sides of a parallelogram are equal and parallel)

AD = CF and AD || CF

(iv) As we had observed that one pair of opposite sides (AD and CF) of quadrilateral

ACFD are equal and parallel to each other, therefore, it is a parallelogram

(v) As ACFD is a parallelogram, therefore, the pair of opposite sides will be equal and parallel to each other

AC || DF and AC = DF

(vi) ΔABC and ΔDEF,

AB = DE (Given)

BC = EF (Given)

AC = DF (ACFD is a parallelogram)

ΔABC ΔDEF (By SSS congruence rule)