In Fig. 9.27, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that: (i) ar (ACB) = ar (ACF) (ii) ar (AEDF) = ar (ABCDE)

Question

Philoid · Accepted Answer

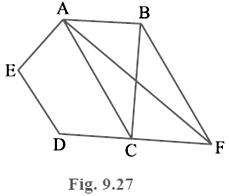
(i) ΔACB and ΔACF lie on the same base AC and are between the same parallels AC and BF

Area (ΔACB) = Area (ΔACF)

(ii) It can be observed that:

Area (ΔACB) = Area (ΔACF)

Area (ΔACB) + Area (ACDE) = Area (ACF) + Area (ACDE)

Area (ABCDE) = Area (AEDF)

In Fig. 9.27, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that:(i) ar (ACB) = ar (ACF)(ii) ar (AEDF) = ar (ABCDE)

In Fig. 9.27, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that:
(i) ar (ACB) = ar (ACF)

(ii) ar (AEDF) = ar (ABCDE)