Let us consider a circle with centre O. Let AB be a tangent which touches the circle at P.

We have to prove that the line perpendicular to AB at P passes through centre O.

Assume that the perpendicular to AB at P does not pass through centre O. Let it pass through another point O'. Join OP and O'P.

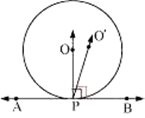
As perpendicular to AB at P passes through O', therefore,

∠ O'PB = 90° ... (1)

O is the centre of the circle and P is the point of contact. We know the line joining the centre and the point of contact to the tangent of the circle are perpendicular to each other.

∴ ∠ OPB = 90° ... (2)

Comparing equations (1) and (2), we obtain

∠ O'PB = ∠ OPB ... (3)

From the figure, it can be observed that,

∠ O'PB < ∠ OPB ... (4)

Therefore, ∠ O'PB = ∠ OPB is not possible. It is only possible, when the line O'P coincides with OP.

Therefore, the perpendicular to AB through P passes through centre O.