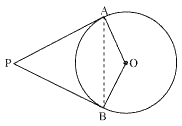
Let us consider a circle centred at point O.

Let P be an external point from which two tangents PA and PB are drawn to the circle which touches the circle at point A and B respectively and AB is the line segment, joining point of contacts A and B together such that it subtends

∠ AOB at centre O of the circle.

It can be observed that

OA ⊥ PA (radius of circle is always perpendicular to tangent)

Therefore, ∠ OAP = 90°

Similarly, OB ⊥ PB

∠ OBP = 90°

In quadrilateral OAPB,

Sum of all interior angles = 360°

∠ OAP +∠ APB+∠ PBO +∠ BOA = 360°

90° + ∠ APB + 90° + ∠ BOA = 360°

∠ APB + ∠ BOA = 180°

Hence, it can be observed that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line-segment joining the points of contact at the centre.