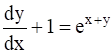
Given Differential equation is:

⇒ ……(1)

Let us assume z = x + y

Differentiate w.r.t x on both sides we get,

⇒

⇒ ……(2)

Substitute(2) in (1) we get,

⇒

Bringing like variables on same side (i.e., variable seperable technique) we get,

⇒

⇒ e^–zdz = dx

Integrating on both sides we get,

⇒ ∫e^–zdz = ∫dx

We know that:

(1) ∫adx = ax + C

(2)

⇒

⇒ –e^–z = x + C

⇒ x + e^–z + C = 0

Since z = x + y we substitute this,

⇒ x + e^{–(x + y)} + C = 0

∴ The solution for the given Differential Equation is x + e^{–(x + y)} + C = 0.