Find the area of the region in the first quadrant bounded by the parabola y = 4x2 and the lines x = 0, y = 1 and y = 4.
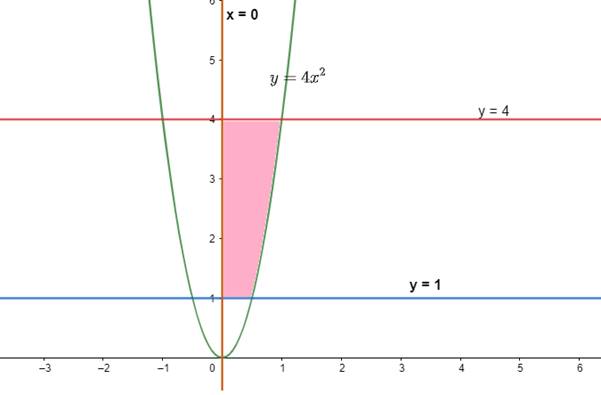
To find the area under two or more than two curves, the first crucial step is to find the INTERSECTION POINTS of the curves.
The coordinates 
⟹ y = 4x2, y = 4
⟹ 4 = 4x2
⟹ x = + 1
Required Area can be calculated by breaking the problem into two parts.
I. Calculate Area under the curve A and Line C
II. Subtract the area enclosed by curve A and Line B from the above area.
Therefore, the areas are:
I. 
= Area enclosed by line C and curve A
II. 
= Area enclosed by curve A and Line B.
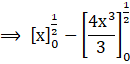
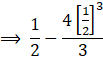
Now the required area under the curves:
1