Draw a rough sketch of the region {(x,y) : y2 ≤ 5x, 5x2 + 5y2 ≤ 36} and find the area enclosed by the region using the method of integration.

To find the area enclosed by the region{(x,y) : y2 ≤ 5x, 5x2 + 5y2 ≤ 36}

The given equations are,


y2 = 5x ...(i)


And 5x2 + 5y2 = 36 ...(ii)



Substituting the value of y2 from (i) into (ii)


5x2 + 25x = 36


5x2 + 25x – 36 = 0


x =


Equation (i) represents a parabola with vertex (0, 0) and axis as x - axis.


Equation (ii) represents a circle with centre (0, 0) and radius 6/√5 and meets axes at and . X ordinate of the point of intersection of circle and parabola is A where a = .


A rough sketch of curves is: -



Required area = Region OCBAO


= 2 (Region OBAO)


= 2 (Region ODAO + Region DBAD)







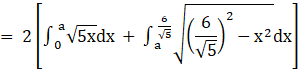
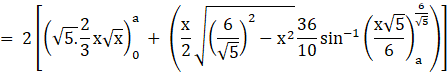
The area enclosed by the region is sq. Units


14