Let point P = (1, 1, 2) and Q be the foot of the perpendicular drawn from P to the plane 2x – 2y + 4z + 5 = 0.

Direction ratios of PQ are proportional to 2, –2, 4 as PQ is normal to the plane.

Recall the equation of the line passing through (x₁, y₁, z₁) and having direction ratios proportional to l, m, n is given by

Here, (x₁, y₁, z₁) = (1, 1, 2) and (l, m, n) = (2, –2, 4)

Hence, the equation of PQ is

⇒ x = 2α + 1, y = –2α + 1, z = 4α + 2

Let Q = (2α + 1, –2α + 1, 4α + 2).

This point lies on the given plane, which means this point satisfies the plane equation.

⇒ 2(2α + 1) – 2(–2α + 1) + 4(4α + 2) + 5 = 0

⇒ 4α + 2 + 4α – 2 + 16α + 8 + 5 = 0

⇒ 24α + 13 = 0

⇒ 24α = –13

We have Q = (2α + 1, –2α + 1, 4α + 2)

Recall the length of the perpendicular drawn from (x₁, y₁, z₁) to the plane Ax + By + Cz + D = 0 is given by

Here, (x₁, y₁, z₁) = (1, 1, 2) and (A, B, C, D) = (2, –2, 4, 5)

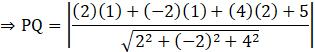
Thus, the required foot of perpendicular is and the length of the perpendicular is units.