Given: - Two equation;

Parabola x = 8 + 2y – y² ,

y - axis,

Line1 y = – 1, and Line2 y = 3

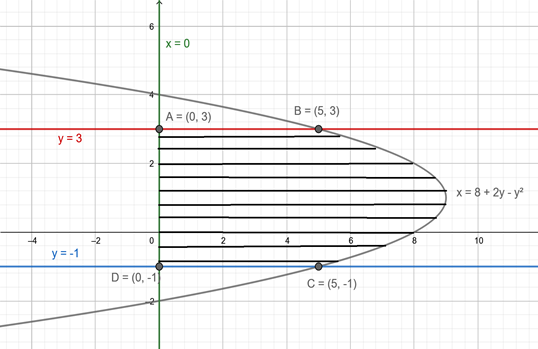
Now to find the area between these four curves, we have to find a common area (ABDC) or the shaded part.

The 1^st intersection of a parabola with line y = – 1, we get,

Putting the value of y = 1 in parabolic equation

⇒ x = 8 + 2y – y²

⇒ x = 8 + 2( – 1) – 1

⇒ x = 5

Hence intersection point is D(5, – 1)

The 2^nd intersection of parabola with y = 3

Putting the value of y in parabola equation

⇒ x = 8 + 2y – y²

⇒ x = 8 + 2(3) – 3²

⇒ x = 8 + 6 – 9

⇒ x = 5

Hence, intersection point is C(5,3)

and other points are A(0,3), B(0, – 1)

From the figure, we can see that, By taking a horizontal strip

The area under shaded portion = Area under parabola from y =– 1 to y = 3.

Tip: - Take limits as per strips. If strip is horizontal than take y limits or if integrating concerning y then limits are of y.

Here, limits are for y i.e. from – 1 to 3

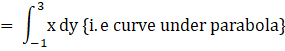
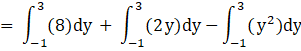
Now putting limits, we get,