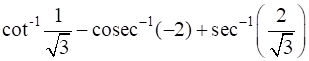
Let cot^–1() = y

⇒ cot y =

= – cot

= cot

= cot

The range of principal value of cot^–1is (0, π)

and cot

∴ The principal value of cot^–1() is …(1)

Let,

cosec^–1–2 ⁼ z

⇒ cosec z = –2

⇒ –cosec z = 2

⇒ –cosec = 2

As we know cosec(–θ) = –cosecθ

∴ –cosec = cosec

The range of principal value of cosec^–1 is –{0} and

cosec = –2

Therefore, the principal value of cosec^–1(–2) is …(2)

Let sec^–1 = w

⇒ sec w =

= sec

The range of principal value of sec^–1is [0, π]–{}

and sec

Therefore, the principal value of sec^–1() is …(3)

From (1), (2) and (3) we can write the above equation as

=

=

=