In Fig. 6.61, two chords AB and CD intersect each other at the point P. Prove that: (i) Δ APC ~ Δ DPB (ii) AP . PB = CP . DP

Question

In Fig. 6.61, two chords AB and CD intersect each other at the point P. Prove that: (i) &#x394; APC ~ &#x394; DPB (ii) AP . PB = CP . DP

Philoid · Accepted Answer

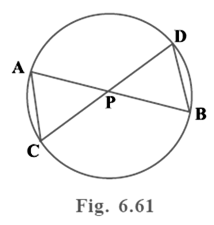
(i) In triangle APC and DPB,

∠CAP = ∠BDP (Angles on the same side of a chord are equal)

∠APC = ∠DPB (Opposite angles)

Hence,

Δ APC ~ Δ DPB (By AAA similarity)

(ii) Since, the two triangles are similar

Hence,

Or,

AP * PB = CP * DP

Hence, proved