Steps of construction:

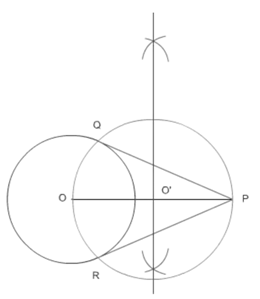
i. Draw a circle with radius 6 cm and centre O.

ii. Now, make a line segment OP = 10 cm

iii. Then, make a perpendicular bisector of OP which intersects OP at O’.

iv. Considering O’P as radius construct another circle.

v. Now, draw tangents to points of intersection between the two circles from point P.

vi. PQ = PR = 8 cm.

Justification: Radius, tangent and distance between centre and external point (from which tangent is made) construct a right triangle. Applying Pythagoras theorem, we have:

PQ² = OP² – OQ²

= 10² – 6²

= 100 – 36 = 64

Or, PQ = 8 cm