Book: RD Sharma - Mathematics (Volume 1)
Chapter: 9. Continuity
Subject: Maths - Class 12th
Q. No. 10 of Exercise 9.1
Listen NCERT Audio Books to boost your productivity and retention power by 2X.
Discuss the continuity of the following functions at the indicated point(s).
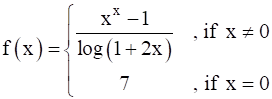at x = 0
In this problem we need to check continuity at x = 0
Given function is
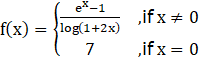at x = 0
∴ we need to check LHL, RHL and value of function at x = 0 (for idea and meaning of continuity refer to Q10(i))
1. NOTE : Idea of logarithmic limit and exponential limit –
……equation 1
…… equation 2
You must have read such limits in class 11. You can verify these by expanding log(1+x) and ex in its taylor form.
Numerator and denominator conditions also hold for this limit like sandwich theorem.
E.g : 
But , 
Now we are ready to solve the problem.
Given function is
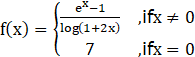at x = 0 …… Equation 3
Clearly,
f(0) = 7 [from equation 2]
LHL = 
[ putting x = –h in equation 3]
= 
Using logarithmic and exponential limit as explained above, we have:
LHL =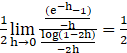 
RHL = 
[ putting x = h in equation 3]
= 
Using logarithmic and exponential limit as explained above, we have:
RHL =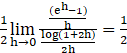 
Thus, LHL = RHL ≠ f(0)
∴ f(x) is discontinuous at x = 0
