For what value of k is the function 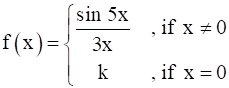 continuous at
continuous at
x = 0?
Ideas required to solve the problem:
1. Meaning of continuity of function – If we talk about a general meaning of continuity of a function f(x) , we can say that if we plot the coordinates (x , f(x)) and try to join all those points in the specified region, we can do so without picking our pen i.e you will put your pen/pencil on graph paper and you can draw the curve without any breakage.
Mathematically we define the same thing as given below:
A function f(x) is said to be continuous at x = c where c is x–coordinate of the point at which continuity is to be checked
If:–
![]() equation 1
equation 1
where h is a very small positive no (can assume h = 0.00000000001 like this )
It means :–
Limiting value of the left neighbourhood of x = c also called left hand limit LHL ![]() must be equal to limiting value of right neighbourhood of x= c called right hand limit RHL
must be equal to limiting value of right neighbourhood of x= c called right hand limit RHL ![]() and both must be equal to the value of f(x) at x=c f(c).
and both must be equal to the value of f(x) at x=c f(c).
Thus, it is the necessary condition for a function to be continuous
So, whenever we check continuity we try to check above equality if it holds true, function is continuous else it is discontinuous.
2. Idea of sandwich theorem – This theorem also known as squeeze theorem that you may have encountered in your class 11 in limits chapter suggests that
If I be an interval having the point a as a limit point. Let g, f, and h be functions defined on I, except possibly at a itself. Suppose that for every x in I not equal to a, we have{displaystyle lim _{x→ a}g(x)=lim _{x→ a}h(x)=L.}{displaystyle lim _{x→ a}f(x)=L.}
g(x) ≤ f(x) ≤ h(x) and also
![]()
Then , ![]() We say that f(x) is squeezed between g(x) and h(x) or you can assume it like sandwich.
We say that f(x) is squeezed between g(x) and h(x) or you can assume it like sandwich.
![]()
∴ ![]() …… equation 2
…… equation 2
NOTE : denominator in the above limit should be exactly same as that of content in sine function
Eg : ![]()
Given,
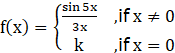 …….equation 3
…….equation 3
We need to find the value of k such that f(x) is continuous at x = 0
Since f(x) is continuous at x = 0
∴ (LHL as x tends to 0) = (RHL as x tends to 0) = f(0)
∴ ![]()
As, f(0) = k [from equation 2]
We can find either LHL or RHL to equate with f(1)
Let’s find RHL, you can proceed with LHL also.
RHL = ![]()
= ![]()
As, f(x) is continuous
∴ RHL = f(0)
∴ k = ![]()