Book: RD Sharma - Mathematics (Volume 1)
Chapter: 9. Continuity
Subject: Maths - Class 12th
Q. No. 42 of Exercise 9.1
Listen NCERT Audio Books to boost your productivity and retention power by 2X.
For what value of is the function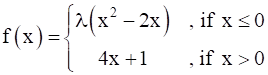 
continuous at x = 0? What about continuity at x = ± 1?
we have to find the value of '
' such that f(x) is continuous at x = 0
If f(x) is be continuous at x = 0,then, f(0)–=f(0) + =f(0)
LHL = f(0)–= 
⇒ 
(02 + 2×0)
⇒ 0 ...(1)
RHL = f(0) + = 
⇒ 4(0) + 1
⇒ 1 ...(2)
From (1) & (2),we get f(0)–=f(0) + ,
Hence f(x) is not continuous at x = 0
we also have to find out the continuity at point 
For f(x) is be continuous at x = 1,
then ,f(0)–=f(0) + =f(0)
LHL = f(1) + = 
⇒ 
(02–1)
⇒ –
...(1)
RHL = f(1) + = 
⇒ (5 + 4×0)
⇒ 5 ...(2)
From (1) & (2),we get f(0)– = f(0) + ,
i.e, –
= 5
⇒ 
= –5
Hence f(x) is continuous at x = 1,when 
= –5
Similarly, For f(x) is be continuous at x = –1,
then ,f(–1)–=f(–1) + =f(–1)
LHL = f(–1)–=
⇒ –
(02 + 4×0 + 3)
⇒ –3 
...(3)
RHL = f(–1) + =
⇒ (–3 + 4×0)
⇒ –3 ...(2)
From (1) & (2),we get, f(–1)–=f(–1) +
i.e, –3
= –3
⇒ 
= 1
Hence f(x) is continuous at x = 1,when 
= 1
