Book: RD Sharma - Mathematics (Volume 1)
Chapter: 9. Continuity
Subject: Maths - Class 12th
Q. No. 3 of Exercise 9.2
Listen NCERT Audio Books to boost your productivity and retention power by 2X.
Find the points of discontinuity, if any, of the following functions :
Basic Idea:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if :
where h is a very small ‘+ve’ no.
i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
This is very precise, using our fundamental idea of limit from class 11 we can summarise it as, A function is continuous at x = c if :
Here we have,
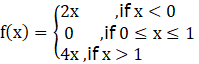……equation 1
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined )
Let c is any random number such that c < 0 [thus c being a random number, it can include all numbers less than 0]
f(c) = 
[ using eqn 1]
Clearly, 
∴ We can say that f(x) is continuous for all x < 0
As x = 0 is a point at which function is changing its nature, so we need to check the continuity here.
f(0) = 0
[using eqn 1]
LHL = 
RHL = 
Thus LHL = RHL = f(0)
∴ f(x) is continuous at x = 0
Let m is any random number such that 0 < m < 1 [thus m being a random number, it can include all numbers greater than 0 and less than 1]
f(m) = 
[ using eqn 1]
Clearly, 
∴ We can say that f(x) is continuous for all 0 < x < 1
As x = 1 is again a point at which function is changing its nature, so we need to check the continuity here.
f(1) = 0
[using eqn 1]
LHL = 
RHL = 
Thus LHL ≠ RHL
∴ f(x) is discontinuous at x = 1
Let k is any random number such that k > 1 [thus k being a random number, it can include all numbers greater than 1]
f(k) = 
[ using eqn 1]
Clearly, 
∴ We can say that f(x) is continuous for all x > 1
Hence, f(x) is continuous for all real value of x, except x =1
There is a single point of discontinuity at x = 1
