Book: RD Sharma - Mathematics (Volume 1)
Chapter: 9. Continuity
Subject: Maths - Class 12th
Q. No. 18 of Exercise 9.2
Listen NCERT Audio Books to boost your productivity and retention power by 2X.
Given the function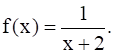 
Find the points of discontinuity of the function f(f(x)).
Basic Idea:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if :
where h is a very small ‘+ve’ no.
i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
This is very precise, using our fundamental idea of limit from class 11 we can summarise it as, A function is continuous at x = c if :
NOTE: If f and g are two functions whose domains are same and both f and g are everywhere continuous then f/g is also everywhere continuous for all R except point at which g(x) = 0
As, f(x) = 
Domain of f = { all Real numbers except 2 } = R – {–2}
Clearly it is not defined at x = –2, for rest of values it is continuous everywhere
Because 1 is everywhere continuous and x + 2 is also everywhere continuous
∴ f(x) is everywhere continuous except at x = –2
f(f(x)) = f
Domain of f(f(x)) = R – {–2 , (
}
For rest of values it just a fraction of two everywhere continuous function
∴ at all other points it is everywhere continuous.
Hence,
f(f(x)) is discontinuous at x = –2 and x = –5/2
