If y = log (sin x), prove that: 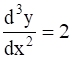 cos x cose3 x.
cos x cose3 x.
Basic idea:
√Second order derivative is nothing but derivative of derivative i.e. ![]()
√The idea of chain rule of differentiation: If f is any real-valued function which is the composition of two functions u and v, i.e. f = v(u(x)). For the sake of simplicity just assume t = u(x)
Then f = v(t). By chain rule, we can write the derivative of f w.r.t to x as:
![]()
√Product rule of differentiation- ![]()
√Apart from these remember the derivatives of some important functions like exponential, logarithmic, trigonometric etc..
Let’s solve now:
As we have to prove: 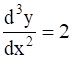 cos x cose3 x
cos x cose3 x
We notice a third order derivative in the expression to be proved so first take the step to find the third order derivative.
Given, y = log (sin x)
Let’s find – ![]()
As ![]()
So lets first find dy/dx and differentiate it again.
∴ ![]()
differentiating ![]() using the chain rule,
using the chain rule,
let, t = sin x and y = log t
∵ ![]() [using chain rule]
[using chain rule]
![]()
[∵ ![]() =
= ![]() &
& ![]() ]
]
![]()
Differentiating again with respect to x :
![]()
![]()
[ ∵ ![]() ]
]
![]()
Differentiating again with respect to x:
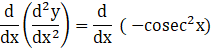
using the chain rule and ![]()
![]()
![]() [ ∵ cot x = cos x/sin x]
[ ∵ cot x = cos x/sin x]
![]()