Note: y₂ represents second order derivative i.e. and y₁ = dy/dx

Given,

x = sin

y = ……equation 1

to prove: (1–x²)y₂–xy₁–a² y = 0

We notice a second order derivative in the expression to be proved so first take the step to find the second order derivative.

Let’s find

As,

So, lets first find dy/dx

∵ y =

Let t = asin^–1 x => []

And y = e^t

…….equation 2

Again differentiating with respect to x applying product rule:

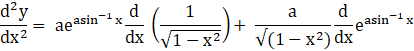
Using chain rule and equation 2:

[using ]

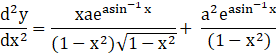
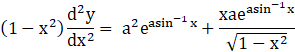
Using equation 1 and equation 2 :

∴ (1–x²)y₂–xy₁–a²y = 0……proved