A manufacturer produces two type of steel trunks. He has two machines A and B. For completing, the first types of the trunk requires 3 hours on machine A and 3 hours on machine B, whereas the second type of the trunk requires 3 hours on machine A and 2 hours on machine B . Machines A and B can work at most for 18 hours and 15 hours per day respectively. He earns a profit of Rs 30 and Rs 25 per trunk of the first type and the second type respectively. How many trunks of each type must
he make each day to make maximum profit?
Let x trunks of first type and y trunks of second type were manufactured. Number of trunks cannot be negative.
Therefore, x, y 
0
According to the question, the given information can be tabulated as
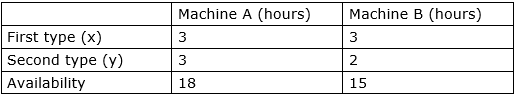
Therefore, the constraints are,
3x + 3y 
18
3x + 2y 
15.
He earns a profit of Rs 30 and Rs 25 per trunk of the first type and the second type respectively. Therefore, profit gained by him from x trunks of first type and y trunks of second type is Rs 30x and Rs 25y respectively.
Total profit Z = 30x + 25y which is to be maximized.
Thus, the mathematical formulation of the given LPP is
Max Z = 30x + 25y
Subject to
3x + 3y 
18
3x + 2y 
15
x, y 
0
Region 3x + 3y 
18: line 3x + 3y = 18 meets axes at A(6,0), B(0,6) respectively. Region containing origin represents the solution of the inequation 3x + 3y 
18 as (0,0) satisfies 3x + 3y 
18.
Region 3x + 2y 
15: line 3x + 2y = 15 meets axes at C(5,0), D(0,
) respectively. Region containing origin represents the solution of the inequation 3x + 2y 
15 as (0,0) satisfies 3x + 2y 
15.
Region x,y 
0: it represents first quadrant.
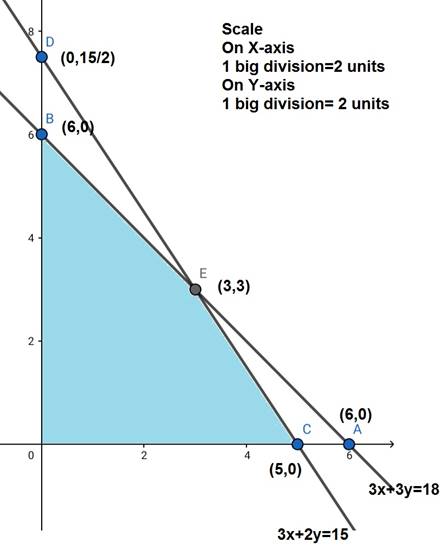
The corner points are O(0,0), B(0,6), E(3,3), and C(5,0).
The values of Z at these corner points are as follows:
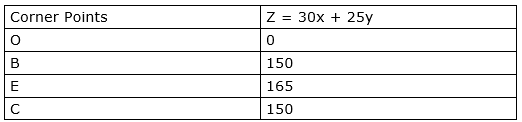
The maximum value of Z is 165 which is attained at E(3,3).
Thus, the maximum profit is of Rs 165 obtained when 3 units of each type of trunk is manufactured.