What is the sum of the measures of the angles of a convex quadrilateral? Will this property hold if the quadrilateral is not convex? (Make a non-convex quadrilateral and try!)

Question

Philoid · Accepted Answer

The angle sum of a convex quadrilateral is 360° as a convex quadrilateral is made of two triangles:

So, 2 × 180° = 2 × 180° = 360°

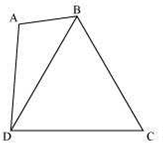
Here,

ABCD is a convex quadrilateral, made of two triangles ΔABD and ΔBCD.

Therefore,

The sum of all the interior angles of this quadrilateral will be same as the sum of all the interior angles of these two triangles i.e.,

180° + 180° = 360°

Yes, this property also holds true for a quadrilateral which is not convex. This is because any quadrilateral can be divided into two triangles

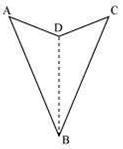
Again, ABCD is a concave quadrilateral, made of two triangles ΔABD and ΔBCD.

Therefore,

Sum of all the interior angles of this quadrilateral will be:

= 180° + 180°

= 360°