Consider the following parallelogram. Find the values of the unknowns x, y, z
(i) 
(ii) 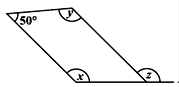
(iii) 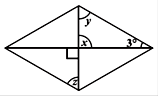
(iv) 
(v) 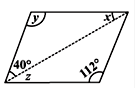
(i) x + 100° = 180° (Adjacent angles are supplementary)
x = 80°
z = x = 80o (Opposite angles are equal)
y = 100° (Opposite angles are equal)
(ii) x, y and z will be complimentary to 500
Hence, required angle = 180 -50
= 1300
x = y = 130° (Opposite angles are equal)
z = x = 130o (Corresponding angles)
(iii) x = 90° (Vertically opposite angles)
x + y + 30° = 180° (Angle sum property of triangles)
120° + y = 180°
y = 60°
z = y = 60° (Alternate interior angles)
(iv) z = 80° (Corresponding angles)
y = 80° (Opposite angles are equal)
x+ y = 180° (Adjacent angles are supplementary)
x = 180° − 80°
= 100°
(v) y = 112° (Opposite angles are equal in a parallelogram)
x+ y + 40° = 180° (Angle sum property of triangles)
x + 112° + 40° = 180°
x + 152° = 180°
x = 28°
z = x = 28° (Alternate interior angles)