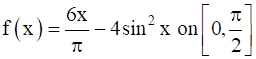
First, let us write the conditions for the applicability of Rolle’s theorem:

For a Real valued function ‘f’:

a) The function ‘f’ needs to be continuous in the closed interval [a,b].

b) The function ‘f’ needs differentiable on the open interval (a,b).

c) f(a) = f(b)

Then there exists at least one c in the open interval (a,b) such that f’(c) = 0.

Given function is:

⇒

We know that sine function is continuous and differentiable over R.

Let’s check the values of function ‘f’ at the extremums,

⇒

⇒ f(0) = 0 – 4(0)

⇒ f(0) = 0

⇒

⇒

⇒

⇒

⇒ .

We got . So, there exists a cϵ such that f’(c) = 0.

Let’s find the derivative of function ‘f.’

⇒

⇒

⇒

⇒

⇒

We have f’(c) = 0

⇒

⇒

⇒

We know

⇒

⇒

⇒

⇒

∴ Rolle’s theorem is verified.