Evaluate the integral:
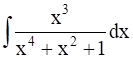
Let, I = ![]()
I = ![]()
If we assume x2 to be an another variable, we can simplify the integral as derivative of x2 i.e. x is present in numerator.
Let, x2 = u
⇒ 2x dx = du
⇒ x dx = 1/2 du
∴ I = ![]()
As, ![]()
∴ Let, u = A(2u + 1) + B
⇒ u = 2Au + A + B
On comparing both sides –
We have,
2A = 1 ⇒ A = 1/2
A + B = 0 ⇒ B = –A = –1/2
Hence,
I =![]()
∴ I = ![]()
Let, I1![]() and I2 =
and I2 = ![]()
Now, I = I1 + I2 ….eqn 1
We will solve I1 and I2 individually.
As, I1 = ![]()
Let v = u2 + u + 1 ⇒ dv = (2u + 1)du
∴ I1 reduces to ![]()
Hence,
I1 = ![]() {∵
{∵ ![]() }
}
On substituting value of u, we have:
I1 = ![]() ….eqn 2
….eqn 2
As, I2 = ![]() and we don’t have any derivative of function present in denominator. ∴ we will use some special integrals to solve the problem.
and we don’t have any derivative of function present in denominator. ∴ we will use some special integrals to solve the problem.
As denominator doesn’t have any square root term. So one of the following two integrals will solve the problem.
![]()
Now we have to reduce I2 such that it matches with any of above two forms.
We will make to create a complete square so that no individual term of x is seen in denominator.
∴ I2 = ![]()
⇒ I2 = ![]()
Using: a2 + 2ab + b2 = (a + b)2
We have:
I2 =![]()
∴ I2 = 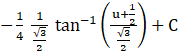
∴ ![]() …eqn 3
…eqn 3
From eqn 1, we have:
I = I1 + I2
Using eqn 2 and 3, we get –
I = ![]()
Putting value of u in I:
I = ![]()
I = ![]()