Let f(x) =

Substituting x = – x in f(x)

f( – x) =

f(x) = f( – x)

it is an even function

………(1)

Now,

f(x) = |x cosπx| = x cosπx; for x [0,12]

= – x cosπx; for x [1/2,1]

Using interval addition property of integration, we know that

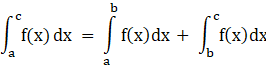
Equation 1 can be written as

2[]

Putting the limits in above equation

= 2{[(x/π)sinx + (1/π²)cosπx]₀^1/2 – [(x/πsinx + (1/π²)cosπx]¹_1/2}

= 2{[(1/2π) – (1/π²)] – [( – 1/π²) – (1/2π)]}

= 2/π