Book: RD Sharma - Mathematics (Volume 2)
Chapter: 33. Binomial Distribution
Subject: Maths - Class 12th
Q. No. 27 of Exercise 33.1
Listen NCERT Audio Books to boost your productivity and retention power by 2X.
Five dice are thrown simultaneously. If the occurrence of 3, 4 or 5 in a single die is considered a success, find the probability of at least 3 successes.
Let p denote the probability of getting 3, 4 or 5 in a throw of dice.
Let us find out the value of p.
We know, a dice has 6 faces numbered 1, 2, 3, 4, 5 and 6.
So, the probability of getting a 3, 4 or 5 is given as,
If p denotes the probability of getting success, then let q denote the probability of not getting success.
We can say,
p + q = 1
⇒ q = 1 – p
Let X denote the number of successes in the throw of five dice simultaneously.
Let there be total n number of throws of five dice simultaneously.
Then, the probability of getting r successes out of n throws of dice is given by,
P (X = r) = nCrprqn-r
Now, substitute the value of p and q in the above equation.
Also, put n = 5 (Since there are 5 dice throw)
Now, the probability of getting at least 3 successes is given by
Probability of getting at least 3 successes = P(X = 3) + P(X = 4) + P(X = 5)
Thus,
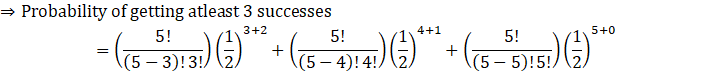
Thus, the probability of getting 3 successes is 1/2.
