If f(x) = loge(1 – x) and g(x) = [x], then determine each of the following functions:
i. f + g
ii. fg
iii. 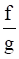
iv. 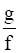
Also, find (f + g)(–1), (fg)(0), ![]() and
and ![]() .
.
Given f(x) = loge(1 – x) and g(x) = [x]
Clearly, f(x) takes real values only when 1 – x > 0
⇒ 1 > x
⇒ x < 1
∴ x ∈ (–∞, 1)
Thus, domain of f = (–∞, 1)
g(x) is defined for all real numbers x.
Thus, domain of g = R
i. f + g
We know (f + g)(x) = f(x) + g(x)
∴ (f + g)(x) = loge(1 – x) + [x]
Domain of f + g = Domain of f ∩ Domain of g
⇒ Domain of f + g = (–∞, 1) ∩ R
∴ Domain of f + g = (–∞, 1)
Thus, f + g : (–∞, 1) → R is given by (f + g)(x) = loge(1 – x) + [x]
ii. fg
We know (fg)(x) = f(x)g(x)
⇒ (fg)(x) = loge(1 – x) × [x]
∴ (fg)(x) = [x]loge(1 – x)
Domain of fg = Domain of f ∩ Domain of g
⇒ Domain of fg = (–∞, 1) ∩ R
∴ Domain of fg = (–∞, 1)
Thus, f – g : (–∞, 1) → R is given by (fg)(x) = [x]loge(1 – x)
iii. ![]()
We know ![]()
![]()
As earlier, domain of ![]() = (–∞, 1)
= (–∞, 1)
However,![]() is defined for all real values of x ∈ (–∞, 1), except for the case when [x] = 0.
is defined for all real values of x ∈ (–∞, 1), except for the case when [x] = 0.
We have [x] = 0 when 0 ≤ x < 1 or x ∈ [0, 1)
When 0 ≤ x < 1, ![]() will be undefined as the division result will be indeterminate.
will be undefined as the division result will be indeterminate.
⇒ Domain of ![]() = (–∞, 1) – [0, 1)
= (–∞, 1) – [0, 1)
∴ Domain of ![]() = (–∞, 0)
= (–∞, 0)
Thus, ![]() : (–∞, 0) → R is given by
: (–∞, 0) → R is given by ![]()
iv. ![]()
We know ![]()
![]()
As earlier, domain of ![]() = (–∞, 1)
= (–∞, 1)
However,![]() is defined for all real values of x ∈ (–∞, 1), except for the case when loge(1 – x) = 0.
is defined for all real values of x ∈ (–∞, 1), except for the case when loge(1 – x) = 0.
loge(1 – x) = 0 ⇒ 1 – x = 1 or x = 0
When x = 0, ![]() will be undefined as the division result will be indeterminate.
will be undefined as the division result will be indeterminate.
⇒ Domain of ![]() = (–∞, 1) – {0}
= (–∞, 1) – {0}
∴ Domain of ![]() = (–∞, 0) ∪ (0, ∞)
= (–∞, 0) ∪ (0, ∞)
Thus, ![]() : (–∞, 0) ∪ (0, ∞) → R is given by
: (–∞, 0) ∪ (0, ∞) → R is given by ![]()
We have (f + g)(x) = loge(1 – x) + [x], x ∈ (–∞, 1)
We need to find (f + g)(–1).
Substituting x = –1 in the above equation, we get
(f + g)(–1) = loge(1 – (–1)) + [–1]
⇒ (f + g)(–1) = loge(1 + 1) + (–1)
∴ (f + g)(–1) = loge2 – 1
Thus, (f + g)(–1) = loge2 – 1
We have (fg)(x) = [x]loge(1 – x), x ∈ (–∞, 1)
We need to find (fg)(0).
Substituting x = 0 in the above equation, we get
(fg)(0) = [0]loge(1 – 0)
⇒ (fg)(0) = 0 × loge1
∴ (fg)(0) = 0
Thus, (fg)(0) = 0
We have![]() , x ∈ (–∞, 0)
, x ∈ (–∞, 0)
We need to find ![]()
However, ![]() is not in the domain of
is not in the domain of![]() .
.
Thus, ![]() does not exist.
does not exist.
We have![]() , x ∈ (–∞, 0) ∪ (0, ∞)
, x ∈ (–∞, 0) ∪ (0, ∞)
We need to find ![]()
Substituting ![]() in the above equation, we get
in the above equation, we get
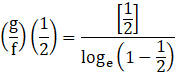
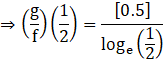

![]()
Thus, ![]()