Given that we need to find the equation of the circle that circumscribes the triangle formed by the lines x = 0, y = 0, and lx + my = 1.

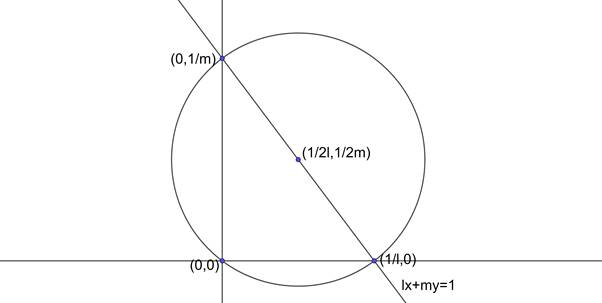
Let us assume A, B, C are the vertices of the triangle.

On solving the lines we get,

⇒ A = (0,0)

⇒ B =

⇒ C =

We have the circle passing through the points A(0,0), B and C.

We know that the standard form of the equation of the circle is given by:

⇒ x² + y² + 2ax + 2by + c = 0 ..... (1)

Substituting A(0,0) in (1), we get,

⇒ 0² + 0² + 2a(0) + 2b(0) + c = 0

⇒ c = 0 ..... (2)

Substituting B in (1), we get,

⇒

⇒

⇒ cm² + 2mb + 1 = 0 ..... - (3)

Substituting C in (1), we get,

⇒

⇒

⇒ cl² + 2al + 1 = 0 ..... (4)

On solving (2), (3) and (4) we get,

⇒

Substituting these values in (1), we get

⇒

⇒

∴The equation of the circle is .