RD Sharma - Mathematics

Book: RD Sharma - Mathematics

Chapter: 7. Values of Trigonometric Functions at Sum of Difference of Angles

Subject: - Class 11th

Q. No. 24 of Exercise 7.1

Listen NCERT Audio Books to boost your productivity and retention power by 2X.

24

If lies in the first quadrant And cos x = 8/17, then prove that

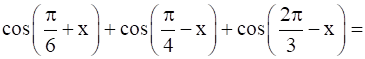
Given x lies in the first quadrant i.e. 0 < x < π/2 And cos x = 8/17


We know that



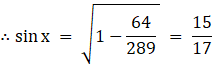
LHS


= cos(30 + x) + cos(45 – x) + cos(120 – x)


We know that cos(A ±B) = cosA cosB ∓ sinA sinB


= cos 30° cos x – sin 30° sin x + cos 45° cos x + sin 45° sin x + cos 120° cos x + sin 120° sin x


= cos x(cos 30° + cos 45° + cos 120°) + sin x(-sin 30° + sin 45° + sin 120°)





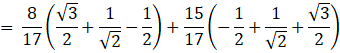
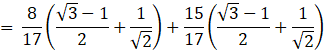
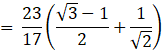
= RHS


Hence, proved.


Chapter Exercises

Exercise 7.1
Exercise 7.2
Very Short Answer
MCQ

More Exercise Questions

1

If sinA = 4/5 And cosB = 5/13, where 0 <A, B < π/2, find the values of the following:

(i) sin(A +B)


(ii) cos(A +B)


(iii) sin(A –B)


(iv) cos(A -B)
2

If SinA = 12/13 And sinB = 4/5, where π/2<A < π And 0 <B < π/2, find the following:

(i) sin(A +B) (ii) cos(A +B)
2

If sinA = 3/5, cosB = –12/13, where A And B Both lie in second quadrant, find the value of sin(A +B).
3

If cosA = – 24/25 And cosB = 3/5, where π <A < 3π/2 And 3π/2 <B < 2π, find the following:

(i) sin(A +B) (ii) cos(A +B)
4

If tanA = 3/4, cosB = 9/41, where π<A < 3π/2 And 0 <B < π/2, find tan(A +B).
5

If sinA = 1/2, cosB = 12/13, where π/2<A < π And 3π/2 <B < 2π, find tan(A -B).
6

If SinA = 1/2, cosB = , where π/2<A < π And 0 <B < π/2, find the following:

(i) tan(A +B)(ii) tan(A -B)
7

Evaluate the following:

(i) sin 780 cos 180 – cos 780 sin 180 (ii) cos 470 cos 130 - sin 470 sin 130


(iii) sin 360 cos 90 + cos 360 sin 90 (iv) cos 800 cos 200 + sin 800 sin 200
8

If cosA = –12/13 and cotB = 24/7, where A lies in the second quadrant and B in the third quadrant, find the values of the following:

(i) sin(A +B) (ii) cos(A +B) (iii) tan(A +B)
9

Prove that: cos 7π/12 + cos π/12 = sin 5π/12 – sin π/12
10

Prove that:

11

Prove that:

(i) (ii)


(ii)

12

Prove that:
12

Prove that:
12

Prove that:
13

Prove that:

14

If tanA = 5/6 And tanB = 1/11, prove thatA +B = π/4.
14

If tanA = m/m–1 And tanB = 1/2m – 1, then prove that A –B = π/4.
15

prove that:

cos2 π/4 - sin2
15

prove that:

sin2(n + 1)A – sin2nA = sin(2n + 1)A sinA
16

Prove that:
16

Prove that:
16

Prove that:
16

Prove that:

sin2B = sin2A + sin2(A-B) – 2sinA cosB sin(A-B)
16

Prove that:

cos2A + cos2B – 2 cosA cosB cos(A +B) = sin2(A +B)
16

Prove that:
17

Prove that:

tan 8x – tan6x – tan 2x = tan 8x


tan 6x tan 2x
17

Prove that:
17

Prove that:

tan 360 + tan 90 + tan 360 tan 90 = 1
17

Prove that:

tan13x – tan 9x – tan 4x = tan 13 x


Tan 9x tan 4x
18

Prove that:

19

If , show that .
20

If tanA = tanB, prove that .
21

If tan(A +B) = And tan(A -B) = y, find the values of tan 2A And tan 2B.
22

If CosA + SinB = m And SinA + CosB = n, prove that 2 Sin(A +B) = m2 + n2 – 2.
23

If tanA + tanB =A And CotA + CotB =B, prove that: cot(A +B) = 1/a – 1/b.
24

If lies in the first quadrant And cos x = 8/17, then prove that
25

If then prove that .
26

If sin(α + β) = 1 And sin(α – β) = 1/2, where , then find the values of tan(α + 2β) And tan(2α + β).
27

If α,β are two different values of x lying between 0 And 2π which satisfy the equation 6 cos x + 8 sin x = 9, find the value of Sin(α+β).
28

If sin α + sin β =A And cos α + cos β =B, show that

(i)


(ii)

29

Prove that:
29

Prove that:
29

Prove that:
30

If sin α sin β - cos α cos β + 1 = 0, prove that 1 + cot α tan β = 0.
31

If tan α = x + 1, tanβ = x - 1, show that 2 cot(α - β) = x2.
32

IfAngle θ is divided into two parts such that the tangents of the one part is λ times the tangent of other, And ϕ is their difference, then show that .
33

If , then show that sin α + cos α = cos x.
34

If α And β are two solutions of the equation Atanx + Bsecx = c, then find the values of sin(α + β).

Students Store