We know that the maximum value of Acosα + Bsinα + c is

c + √(A² +B²)

And the minimum value is c - √(a² +B²).

(i) Given f(x) = 12 sin x – 5 cos x

Here A = -5,B = 12 and c = 0

⇒ -13 ≤ 12 sin x - 5 cos x ≤ 13

Hence, the maximum and minimum values of f(x) are 13 and -13 respectively.

(ii) Given f(x) = 12 cos x + 5 sin x + 4

Here A = 12,B = 5 and c = 4

⇒ -9 ≤ 12 cos x + 5 sin x + 4 ≤ 17

Hence, the maximum And minimum values of f(x) are 17 And -9 respectively.

(iii) Given

We know that sin(A -B) = sinA cosB - cosA sinB

Here

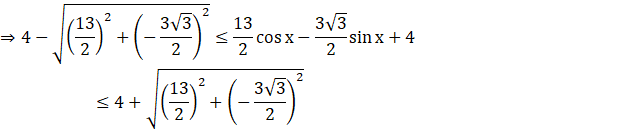
⇒ -3 ≤ ≤ 11

Hence, the maximum And minimum values of f(x) are 11 And -3 respectively.

(iv) Given f(x) = sin x – cos x + 1

Here A = -1,B = 1 And c = 1

Hence, the maximum And minimum values of f(x) are And respectively.