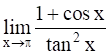
As we need to find

We can directly find the limiting value of a function by putting the value of the variable at which the limiting value is asked if it does not take any indeterminate form (0/0 or ∞/∞ or ∞-∞, .. etc.)

Let Z

∴ we need to take steps to remove this form so that we can get a finite value.

Tip: Similar limit problems involving trigonometric ratios are mostly solved using sandwich theorem.

So to solve this problem we need to have a sin term so that we can make use of sandwich theorem.

Note: While modifying be careful that you don’t introduce any zero terms in the denominator

As,

Multiplying numerator and denominator by 1-cos x, We have-

⇒ Z =

{As 1-cos²x = sin²x}

⇒ Z =

To apply sandwich theorem, we need to have limit such that variable tends to 0 and following forms should be there

Here x→ π so we need to do modifications before applying the theorem.

As, sin (π-x) = sin x or sin (x - π) = -sin x and tan(π – x) = -tan x

∴ we can say that-

sin²x = sin²(x-π) and tan²x = tan²(x-π)

As x → π

∴ (x – π) → 0

Let us represent x - π with y

∴ Z =

Dividing both numerator and denominator by y²

Z =

⇒ Z = 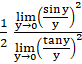{Using basic limits algebra}

As,

∴ Z =

∴