Find the value of k so that the function f is continuous at the indicated point: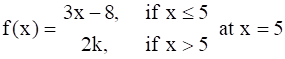
Given,
![]() …(1)
…(1)
We need to find the value of k such that f(x) is continuous at x = 5.
A function f(x) is said to be continuous at x = c if,
Left hand limit(LHL at x = c) = Right hand limit(RHL at x = c) = f(c).
Mathematically we can represent it as-
![]()
Where h is a very small number very close to 0 (h→0)
Now, let’s assume that f(x) is continuous at x = 5.
∴ ![]()
As we have to find k so pick out a combination so that we get k in our equation.
In this question we take LHL = f(5)
∴ ![]()
⇒ ![]() {using equation 1}
{using equation 1}
⇒ 3(5 – 0) – 8 = 2k
⇒ 15 – 8 = 2k
⇒ 2k = 7
∴ k = 7/2
12