Examine the differentiability of f, where f is defined by
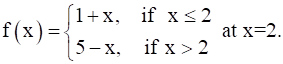
Given,
![]() …(1)
…(1)
We need to check whether f(x) is continuous and differentiable at x = 2
A function f(x) is said to be continuous at x = c if,
Left hand limit(LHL at x = c) = Right hand limit(RHL at x = c) = f(c).
Mathematically we can represent it as-
![]()
Where h is a very small number very close to 0 (h→0)
And a function is said to be differentiable at x = c if it is continuous there and
Left hand derivative(LHD at x = c) = Right hand derivative(RHD at x = c) = f(c).
Mathematically we can represent it as-
![]()
![]()
Finally we can state that for a function to be differentiable at x = c
![]()
Checking for the continuity:
Now according to above theory-
f(x) is continuous at x = 2 if -
![]()
∴ LHL = ![]()
⇒ LHL = ![]() {using equation 1}
{using equation 1}
⇒ LHL = ![]()
∴ LHL = (3-h) = 3
∴ LHL = 3 …(2)
Similarly,
RHL = ![]()
⇒ RHL = ![]() {using equation 1}
{using equation 1}
⇒ RHL = ![]()
∴ RHL = 3+0 = 3 …(3)
And, f(2) = 1 + 2 = 3 {using equation 1} …(4)
From equation 2,3 and 4 we observe that:
![]()
∴ f(x) is continuous at x = 2. So we will proceed now to check the differentiability.
Checking for the differentiability:
Now according to above theory-
f(x) is differentiable at x = 2 if -
![]()
∴ LHD = ![]()
⇒ LHD = ![]() {using equation 1}
{using equation 1}
⇒ LHD = ![]()
∴ LHD = 1 …(5)
Now,
RHD = ![]()
⇒ RHD = ![]() {using equation 1}
{using equation 1}
⇒ RHD = ![]()
∴ RHD = -1 …(6)
Clearly from equation 5 and 6,we can conclude that-
(LHD at x=2) ≠ (RHD at x = 2)
∴ f(x) is not differentiable at x = 2