In a dice game, a player pays a stake of Re1 for each throw of a die. She receives Rs 5 if the die shows a 3, Rs 2 if the die shows a 1 or 6, and nothing otherwise. What is the player’s expected profit per throw over a long series of throws?

Question

Philoid · Accepted Answer

Let X is the random variable of profit per throw.

Probability of getting any number on dice is .

Since, she loss Rs 1 on getting any of 2, 4 or 5.

So, at X= -1,

P(X) = P (2) +P(4) +P(5)

Similarly, =1 if dice shows of either 1 or 6.

P(X) = P (1) +P (6)

and at X=4 if die shows a 3

P(X) = P (3)

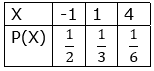
∴ Player’s expected profit = E(X)= XP(X)