Here, S = { (1,2),(1,3),(1,4),(1,5)

(2,1),(2,3),(2,4),(2,5)

(3,1),(3,2),(3,4),(3,5)

(4,1),(4,2),(4,3),(4,5)

(5,1),(5,2),(5,3),(5,4)}

Total Sample Space, n(S) = 20

Let random variable be X which denotes the sum of the numbers on the cards drawn.

∴ X = 3, 4, 5, 6, 7, 8, 9

At X = 3

The cards whose sum is 3 are (1,2), (2,1)

At X = 4

The cards whose sum is 4 are (1,3), (3,1)

At X = 5

The cards whose sum is 5 are (1,4),(2,3),(3,2),(4,1)

At X = 6

The cards whose sum is 6 are (1,5), (2,4),(4,2),(5,1)

At X = 7

The cards whose sum is 7 are (2,5),(3,4),(4,3),(5,2)

At X = 8

The cards whose sum is 8 are (3,5), (5,3)

At X = 9

The cards whose sum is 9 are (4,5), (5,4)

∴ Mean, E(X) = ΣXP(X)

= 6

Also,

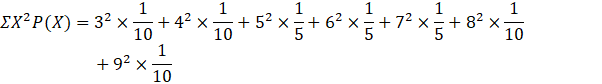
= 39

Now,

Var X = ΣX²P(X) – [ΣXP(X)]²

= 39 – 36

= 3