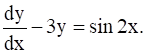
Compare with

we get P = -3 and Q = sin2x

This is linear differential equation where P and Q are functions of x

For the solution of linear differential equation, we first need to find the integrating factor

⇒ IF = e^∫Pdx

⇒ IF = e^∫(-3)dx

⇒ IF = e^-3x

The solution of linear differential equation is given by y(IF) = ∫Q(IF)dx + c

Substituting values for Q and IF

⇒ ye^-3x = ∫e^-3xsin2x dx …. (1)

Let I = ∫e^-3xsin2x dx

If u(x) and v(x) are two functions then by integration by parts,

Here v = sin 2x and u = e^-3x

Applying the above formula, we get,

Again, applying the above stated rule in we get

So,

Put this value in (1) to get,

ye^-3x = ∫e^-3xsin2x dx