Show that the plane ax + by + cz + d = 0 divides the line joining the points (x1, y1, z1) and (x2, y2, z2) in the ratio 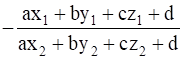 .
.
Given: A(x1, y1, z1) and B(x2, y2, z2)
To prove: the ratio in which the line segment AB is divided by the plane ax + by + cz + d = 0 is ![]()
Formula used:
Section Formula:
A line AB is divided by C in m:n where A(x, y, z) and B(a, b, c).
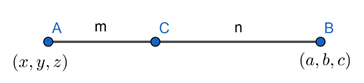
The coordinates of C is given by,
![]()
Let C(x, y, z) be any point on given plane and C divides AB in ratio k: 1

Therefore, m = k and n = 1
A(x1, y1, z1) and B(x2, y2, z2)
Coordinates of C using section formula:
![]()
![]()
On comparing:
![]()
Since, ax + by + cz + d = 0
![]()
![]()
![]()
![]()
![]()
The plane divides AB in the ratio ![]()
Hence Provedco
10