Given: Foci are (6, 4) and (-4, 4) and eccentricity is 2

To find: equation of the hyperbola

Formula used:

The standard form of the equation of the hyperbola is,

Center is the mid-point of two foci.

Distance between the foci is 2ae and b² = a²(e² – 1)

The distance between two points (m, n) and (a, b) is given by

Mid-point theorem:

Mid-point of two points (m, n) and (a, b) is given by

Center of hyperbola having foci (6, 4) and (-4, 4) is given by

= (1, 4)

The distance between the foci is 2ae, and Foci are (6, 4) and (-4, 4)

{∵ e = 2}

b² = a²(e² – 1)

The equation of hyperbola:

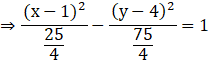
⇒ 12(x² + 1 – 2x) – 4(y² + 16 – 8y) = 75

⇒ 12x² + 12 – 24x – 4y² – 64 + 32y – 75 = 0

⇒ 12x² – 4y² – 24x + 32y – 127 = 0

Hence, required equation of hyperbola is 12x² – 4y² – 24x + 32y – 127 = 0