Given: e₁ and e₂ are respectively the eccentricities of 9x² + 4y² = 36 and 9x² – 4y² = 36 respectively

To find: e₁² – e₂²

9x² – 4y² = 36

Eccentricity(e) of hyperbola is given by,

Here a = 2 and b = 3

Therefore,

For ellipse:

9x² + 4y² = 36

Eccentricity(e) of ellipse is given by,

Here a = 2 and b = 3

Therefore,

Substituting values from (1) and (2) in 2e₁² + e₂²

e₁² – e₂²

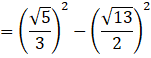
⇒ e₂² – e₂²

Hence, value of 2 < e₂² – e₁² < 3